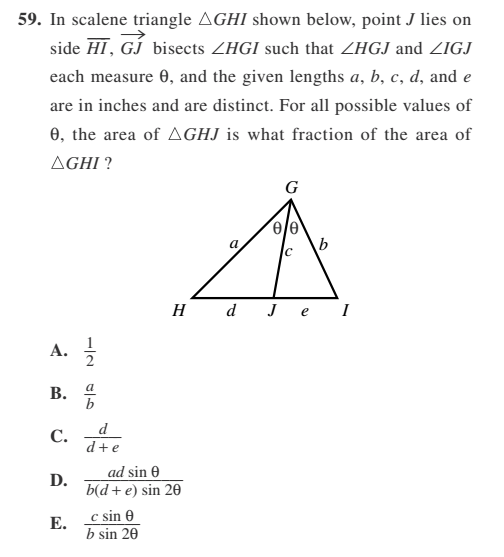
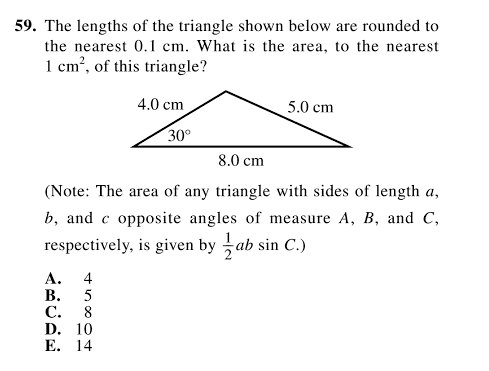
Try this ACT math practice question on rearranging algebraic fractions:
For all values of $x$ where the expression is defined
$$ \dfrac{\dfrac{x-5}{x-2}}{\dfrac{1}{3}-\dfrac{1}{x-2}}=?$$
- $\quad -3$
- $\quad 3$
- $\quad 3(x-5)$
- $\quad \dfrac{x-2}{3(x-5)}$
- $\quad \dfrac{(x-5)^2}{3(x-2)^2}$